
Menu ▼
Camp overview
Kinetics of math is a camp designed to teach students how to spin poi and how to graph those movements using Trigonometry. It is aimed at high school students whom have passed Algebra because familiarity with functions and transformations is necessary. Proficiency is not necessary at this stage. The camp is intended to give students a way to see the connection between things going on in the real world and topics covered in math class.
When in class at school, the focus is so heavily on learning specific skills that it is easy to lose sight of the theme of the topic. At Kinetics in Motion, students will have freedom to play with their ideas and are encouraged to explore with their body, through learning to spin Poi, and explore with their minds, through learning to graph the same material.
Students will spend half of the learning time working increasing their poi skills and half of the learning time playing with math graphing programs. The math portion is intended to be comprehensible, fairly easy, and focused heavily on letting the students explore transformations on the functions to simulate the motions they had experienced in the hands-on poi lessons. The instructors will be on-hand to guide them through any questions they may have about the mathematics and Poi.
See the experience!
During the camp, students switch between 30- to 45-min periods working with Poi and Math. During each period they are taught some lessons then allowed time to play with the concepts, ask questions, and playfully explore what they are working on.
During the Poi lessons, they are given props and are engaged in a fun physical activity. (Who doesn't love spinning things around?)
During the Math lessons, students are guided through topics, such as:
- The Unit Circle
- Quadrants and the Origin
- Angles (Degrees and Radians)
- Polynomial Functions
- Transformations on Functions
- Trigonometric Functions
- Parametric Equations
- How it all connects to Poi Movement
Check out the photos of the students own mathematical artwork:

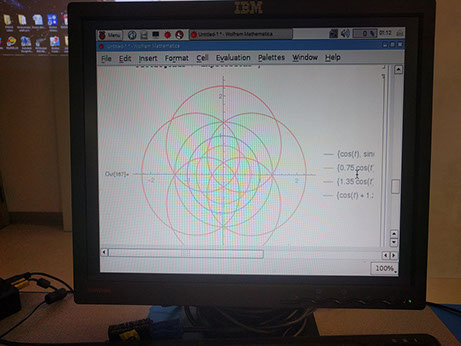
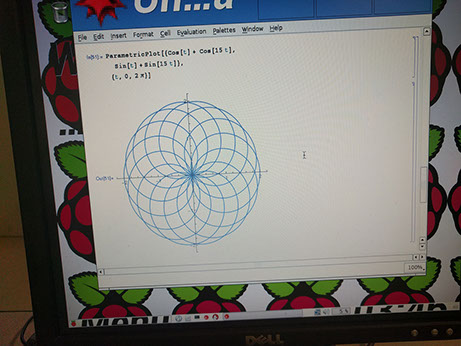
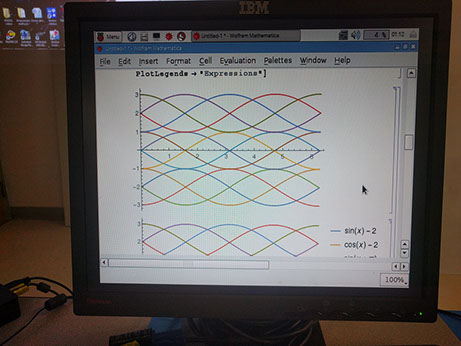




3 - 5
<
>
Biography of Adam
This camp has been designed and will be run by Adam Dipert with some assistance from other instructors.
Adam Dipert is a professional circus artist, and experimental particle and nuclear physics doctoral candidate. He has taught Trigonometry and Algebra at both the college and grade school levels, as well as classical mechanics and electromagnetism. He has worked at and run a plethora of summer camps for students between the ages of 7 to 18.
He received his B.S. in physics from the Ohio State University in 2010 and is working on his Ph. D. at Arizona State University. As an undergraduate, he was given the opportunity to act as a TA for the last 2 years of his degree with his Multivariable Calculus teacher in Trigonometry and PreCalculus courses. He taught 7th grade Algebra for a short time at BASIS Charter School in Tucson Arizona after completing his B.S. but decided to return to the universities shortly after to pursue research. Currently he is studying the depolarization of ³He at 0.3 Kelvin on the Duke University campus at the Triangle Universities Nuclear Laboratory. His advisor is Ricardo Alarcon (ASU). He’s research is being directed by Robert Golub (NCSU), a world leading expert experimentalist in Utracold Neutrons and many other aspects of cryogenics.
He completed high school in 2001 and spent 4 years traveling with Renaissance Festivals, music festivals, county fairs, and was integrated in the circus before his travels ended and he began his studies at Ohio State. Adam worked with Open Air Circus in Somerville MA between 2004-2009 and made significant contributions to the offerings in the program including starting a master class series for older students. The Open Air Circus is a community youth circus focused on students between the ages of 4 to 18. During this time he started working with Grimaldi Circus and Kristina Isabelle Dance Company. Since beginning graduate school, the quality of his circus work has improved significantly and now performs at private, city, and corporate events. He is proficient and frequently performs with Flower Sticks, Contact Juggling, Poi, Ropedart, Ball and Club Juggling, Double Staffs, Diabolo, Stilts, Rolling Globe, and Partner Acrobatics.
Intentions



The intention of this camp is to share the amazing connection between math class and the real world. Too often even math teaches can’t tell you why you should learn a particular set of math skills, Adam can. Mathematics were not invented by nerdy know-it-alls just to make themselves feel better. Mathematics was invented to explain the world. It was invented to give structure to the patterns and ‘chaos’ that is observed in the world around us. It was invented to help organize pattern recognition and communicate these ideas to other people who speak the language.
The most important skill needed to envision the motion of the props is imagination. This is exactly the same skill needed to be successful in mathematics. Students will learn to use their imagination in coordination with their bodies and their mind in productive problem solving situations while having fun and learning to explore the world through the lens of a critical eye trained by convention.
What is poi?
Poi is a form of prop manipulation where a weight is swung at the end of a string. Sounds simple? Nope.
Just like all the best games, the fewer rules you have, the more you can do with it!
The word “Poi” and the first incarnations of this prop come from the Maori tribes of New Zealand. Throughout the last 20 years, a growing number of young people in the Americas and Europe have also started to explore this form of movement. Today people spin poi made out of socks, balls of fire, LEDs, water buckets, bicycle tires, and much more.
Poi will be provided to the students during the workshop. They will be playing with a simple set made out of fabric and rice. About 50% of the instructional time spent at camp will be invested in learning to move with the Poi. We’ll work on basic maneuvers in a plane, antispins, inspins, 2 beat and 3 beat weaves, hybrids, CAPs, and more. (If you don’t understand that terminology, no worries, it’s poi-tech-speak and we’ll understand them by the end.)



SOCK POI
LED POI
FIRE POI
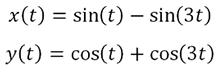
How is poi related to math?
Poi is spun in circles mostly, and that is where our mathematical adventures will begin. Circles can be described simply using polar coordinates. In this coordinate system, an origin is defined and the radius and angle from the origin are the variables. Polar coordinates can be very helpful in some applications but we’ll work in Cartesian Coordinates to get to the real juicy stuff.
Technology
Video from the Raspberry Pi Foundation.
Raspberry Pie is a
delicious treat ...
IT'S ALSO A CREDIT-CARD SIZED COMPUTER THAT IS CAPABLE OF EXTRAORDINARY THINGS.
Raspberry Pi is an open source miniature (credit card sized) computer. They were designed by the Raspberry Pi Foundation intending to have an easy platform for learning the basics of computing. They have done a great job at that! But that’s not why we’re using them. We’re using them because they are easy to transport, easy to clone, easy to network, and come with Mathematica already installed on them! If you’re an educator or computery type then please check out the raspberrypi website.
As mentioned each computer is little and depending upon the version you get, has a few USB ports, an ethernet port, SD card slot for the OS, power plug, HDMI plug, and GPIO for connection to the outside world. We can basically strap them to the back of the monitor, plug a few things in and be ready to go.
Check out the Raspberry Pi website for more info.
The Raspberry Pis (RasPi) which will be used in Kinetics of Math are connected via the Pinet network (replacement for Rasp-LTSP). Pinet is a networking code, designed by an 18 year old who was interested in RasPis being used in classrooms. After he started the code, a number of others contributed on GitHub and before you knew it, the world community had produced a way to allow multiple users to share one closed network on these tiny computers.
For more details, check out the write up here.
Last but not least, the Raspberry Pi is enthusiastically embraced by Stephen Wolfram (the CEO of Wolfram Research and designer of Mathematica and Wolfram Alpha). Mathematica acts somewhat like a very very very very powerful calculator and graphic visualizer for the novice but can be much more than that for the experienced user. It is comparable to MatLab but does not have quite as overwhelming an interface as MatLab. Another similarity to MatLab is that it costs quite a few bucks to have licenses for it (although Wolfram Research does have a great educational outreach program). As mentioned, the aforementioned enthusiast is so enthusiastic about the RasPi, that the RasPi image comes with Mathematica installed... for free! Check out the website if you’re even more curious.
Beyond that... (which already makes perfect sense), Adam has been using Mathematica as part of his research and analysis since his first Classical Mechanics course at Ohio State University 2007. Mathematica can be used to visualize 2D and 3D graphics, with a plethora of options. It can be used to sort and display data from a file if needed. It solves linear equations, ordinary and partial differential equations, can graph parametric equations, and much more. It also has a very simple and easy to understand syntax. This means students will gain some experience with how to accurately follow some rules of syntax without being bogged down with fully learning a software language, our focus will be on the mathematical lessons.
We figured: if we need computers which don’t allow our students to get distracted by video games or internet, and we want to be able to easily transport them to many locations around the country, and we get free Mathematica, let’s go with the Raspberry Pi!